Energy conservation laws
↓
Conservation of energy
↓
Energy cannot be created or destroyed”, energy can only be transformed or converted
(The energy of a isolated system remains constant)
Energy is the capacity or ability to do work
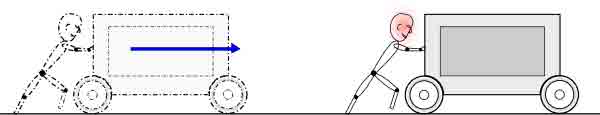
“Work is – moving something against a force”
“Work is done when a force applied to an object causes the object to move”
↓
First law of thermodynamics
For “closed systems” with no external source or sink of energy, the first law of thermodynamics states that a system’s energy is constant unless energy is transferred in or out by mechanical work or heat, and that no energy is lost in transfer.
↓
The change in internal energy of a system is equal to the heat added to the system minus the work carried out by the system
Second law of thermodynamics
All systems doing work always lose some energy as waste heat. This creates a limit to the amount of energy that can do work by a heating process.
↓
Natural processes that involve the transfer or conversion of heat energy are irreversible.
Heat cannot be transferred from a colder object to a hotter object
Basic concepts and units related to work and energy (SI units)
Force – The unit for force is newton (N). 1 newton (N) = 1 kg*1m/s2, which can be translated to the force necessary to accelelrate an object with the mass 1 kg to a velocity of 1 m/second during a period of 1 second.
Work – The unit of work is joule (J). 1 joule = 1 Nm = 1 kg * 1m2/s2, which can be translated to the amount of work performed by a force of 1 newton (N) over a distance of 1 meter in the same direction as the force (or a component of the force).
Energy – The unit for energy is joule (J). 1 joule = 1Nm = 1 kg * 1m2/s2. This is the amount of energy necessary to apply a force of 1 newton over a distance of 1 meter.
Power – The unit for power is watt (W). 1 watt (W) = 1J/s = 1Nm/s = 1 kg * 1m2/s3 or the power produced by a current of one ampere acting across a potential difference of one volt. This can be translated to the amount of work performed per second or the rate of using energy.
A force can do work
↓
Energy is needed to be able to provide the force such that the work can be done
↓
Power is the amount of work carried out per unit of time or the amount of energy needed to do the work per unit of time.
Relative terms
Since energy is defined as the ability to do work on objects, there is no absolute measure of energy. Only the transition of a system from one state to another can be defined and thus energy is measured in relative terms. The choice of a baseline or zero point is often arbitrary and can be made in whatever way that is most convenient for a problem.
Energy density is a term used for the amount of useful energy stored in a system per unit volume. For fuels, the energy per unit volume is a useful parameter to compare fuel effectiveness.
Some forms of energy
| Type | Description |
| Kinetic | Is the energy of motion. The work needed to accelerate a body of a given mass from rest to its stated velocity. |
| Potential | Is stored energy. Potential energy is the energy that an object has due to its position in a force field or that a system has due to the configuration of its parts. |
| Chemical | Is the energy contained in molecules. |
| Electric | Is energy from electric fields. |
| Magnetic | Is energy from magnetic fields. |
| Nuclear | Is the energy of binding nucleons to form the atomic nucleus. |
| Heat | The amount of thermal energy being transferred (in a given process) during or as a consequence of changed temperature. |
| Mechanical work | The amount of energy being transferred (in a given process) due to motion of an object in the direction of an applied force. |
Mass-energy equivalence
The mass equivalent theory formulated by Albert Einstein describes the relationship between energy and mass by the following equation:
E=mc^2
It also defines that objects at rest has a fundamental energy to them, a rest energy E_0 , which is:
E_0=m_0c^2
The rest energy E_0=m_0c^2 – Is the energy it has because it exist.
The rest energy is the internal energy of an object, particle or system with a certain mass, that is at rest:
and
Ek= Kinetic energy – Is the energy it has because it is moving.
E=mc^2
or
E= m_0c^2 + E_k
Is the full energy or the relativistic energy, describing the total energy of an object, particle or system.
Therefore
E_k = mc^2 - m_0c^2
where:
m = relativistic (real) mass
m0= rest mass
c = speed of light (in vacuum)
The mass energy equivalence demonstrates that mass and energy is closely tied together.
If the rest energy of an object increases also the mass of the object will increase since the speed of light (“c”) in vacuum is constant.
If the object is an elastic spring and it is stretched or compressed, its potential energy increases, the internal energy of the spring, E0 has grown slightly and thus also the spring mass m0 becomes larger.
If, for example, the potential energy of an object of 1 kg is increased by 1 kJ the mass of the object would increase by:
dE_0=dm_0c^2
dm_0 = \dfrac{dE_0}{C^2}= \dfrac{1000J}{299792458 \space m/s}
= 0,000000000000011 \space kg
= 1,1 *10^{-14} \space kg \space
= 1,1 *10^{-11} \space g
Energy is always preserved, but can manifest in different forms. It may be as radiation, potential or chemical energy or as mass.
Using the mass-energy equivalence, we can find out, as an example, how much energy a piece of wood of 1 kg corresponds to.
E_0=m_0c^2
= 1 Kg * (299792458 m / s )2
= 8,98755178 1013 kJ
= 8,98755178 TJ.
= 89,8755178 Petajoules
= 24,9654216 Million kWh
= 21,4663986 Trillion kcal
= 85,1855545 Trillion Btu
In comparison the same piece of wood would release around 14500 KJ if burned as firewood (provided complete combustion).
For gasoline the corresponding energy released when burning 1 kg would be around 44400 KJ.
in other words the rest energy, if it could be utilised, of a mass of 1 kg represents the same amount of energy as burning 2,005 Billion kg of gasoline or 2,673 Billion litres of gasoline.
The vast amount of energy in the form of rest mass can not yet be used for practical purposes.
Even in nuclear processes only a small fraction of the rest energy may be released.
Energy of Light
Does light have mass?
No, photons do not have mass (rest mass).
Light has energy even if it does not have mass.
Let us have a look at the implications of calculating the energy of light:
E=mc^2
and
E= \gamma m_0c^2
where
\gamma =\dfrac{1}{\sqrt{1-(v/c)^2}} (Lorentz factor)
(Lorentz factor is the factor used within special relativity, by which time, length, and relativistic mass change for an object while that object is moving)
From the above expressions we see that anything with a mass would have infinite energy if it travelled at the speed of light, v = c.
\gamma =\dfrac{1}{\sqrt{1-(c/c)^2}} \rightarrow ∞
The reason that light does not have infinite energy is because it has zero rest mass.
Since m0 for a photon (light) is zero and \gamma (at the speed of light) is infinite we cannot determine the energy of light directly by the equation E = mc2, since the result of zero times infinite would be unknown.
We therefore need to do “go another route” to calculate the energy of a proton (light).
From
E= \gamma m_0c^2
we get
\gamma m_0 = \dfrac{E}{c^2}
We also know that the momentum “p” of a particle is the product of its mass times its velocity
p =mv
At relativistic velocities (speed of light or close to the speed of light) we need to apply the relativistic mass m =m_0\gamma .
p = \gamma m_0v
by combining the expressions above we get:
p = \dfrac{E}{c^2}v = \dfrac{E}{c^2}c = \dfrac{E}{c}
or
E= pc
we also know, according to Einstein, that the energy of a photon is the frequency times Planck constant:
E= hf
which gives us:
p = \dfrac{hf}{c}
Since light is electromagnetic waves and the speed of a wave may be expressed as the frequency times the wave lenght:
v= f \lambda
and for light
v= c
therefore
c= f \lambda
that means:
\dfrac{1}{\lambda} = \dfrac{f}{c}
which makes it possible to express the momentum of a photon by the frequency and the wave lenght
p = \dfrac{f}{\lambda}
h = Planck constant
f = frequency
\lambda = Wave lenght
Since photons have momentum they can move things.
Solar sails, using the radiation pressure from sunlight onto large mirrors for spacecraft propulsion is one example of utilising the momentum of photons.
Another example, theoretical though, is If you were holding an extremely powerful flash light as it was switched on, you would be “pushed” back with the same force as and in the opposite direction of the light.
Light has energy even if it does not have mass.
To learn more about electromagnetic energy please go to: “electromagnetic energy”